Is there art in number?
SquareCirclez Mathematics, learning, computing, travel - and whatever...
- The Ultimate Guide to AI Math Solvers: Boosting Your Math Skills with Technologyby Casey Allen on February 6, 2025 at 4:37 am
The Ultimate Guide to AI Math Solvers: Boosting Your Math Skills with Technology Introduction to AI Math Solvers Mathematics has always been a subject that challenges students, often requiring extra practice and assistance to master. With the rapid advancements in technology, Artificial Intelligence (AI) has stepped in to bridge the gap, offering innovative solutions that Related posts: 5 Best Free Math Problem Solvers Math problems allow students to learn new concepts and strengthen... Math Homework Help: A Guide to the Best AI Math Solver of 2025 About a quarter of the average college student's courseload is... Solutions: A Guide to Problem Solving Review: Paperback This book is about general problem solving ('in... 21st century math skills A reader asks for advice on 21st century skills and...
- Math Homework Help: A Guide to the Best AI Math Solver of 2025by Casey Allen on December 1, 2024 at 4:20 am
About a quarter of the average college student's courseload is general education requirements. While these are graduation requirements, they also are usually time-wasters. They're challenging and stressful... but luckily, help is available. If you're looking for quick math homework help, an online AI math solver can bring your grades up quickly and effectively. Read on to Related posts: 5 Best Free Math Problem Solvers Math problems allow students to learn new concepts and strengthen... My dilemma - ethical math help Is there a difference between paying someone to do... Buyer’s Guide: TI-84 Graphing Calculator Math classes can be daunting. From a young age, I... Curriculum Webs - more homework needed "Weaving the Web into Teaching and Learning" Cunningham, C and...
- 5 Best Free Math Problem Solversby Casey Allen on June 6, 2023 at 3:43 am
Math problems allow students to learn new concepts and strengthen problem-solving skills. But many learners feel confused or frustrated if they can’t find the correct solution. A math problem solver is a handy tool that helps students doublecheck their work and identify errors. However, not all math problem solvers are created equal. Here are the Related posts: Microsoft Math 3.0 Review MS Math 3.0 is a well-designed computer-based math tool.... Free math software downloads Wanting to use some math software but find it’s too... GraphSketch.com - free online math grapher GraphSketch is a free offering that allows the user to... Context Free math-based art Context Free is software you can use to produce some...
- Reviewing Six Online Math Tutoring Services - What’s the Best?by Hugo Pegley on June 22, 2022 at 4:00 am
Math is an exciting field of study that can lead to a variety of exciting careers or research projects. But if you're a student having difficulty with the topic, you might be thinking about enrolling in an online math tutoring program. This is a great way for you to get assistance in a format and Related posts: How to Pick A Live Math Chat Tutoring Service If you’re looking for a live math tutor, you are... How Much Does an Online Math Tutor Cost? Across the world, math is the key to understanding many... Online Algebra Math Tutor Many private and public high schools and colleges require students... Best Online Calculus Math Tutor: How to Choose Calculus and math require tremendous background information, practice, and good...
- Picking the Best Online Precalculus Math Tutorby Hugo Pegley on June 22, 2022 at 3:55 am
Students who want to go on to study math, science, engineering, and other disciplines in college, usually find that their chosen college values some prior knowledge of calculus. An online precalculus math tutor could be the answer. High schools commonly offer precalculus courses in the 11th grade before introducing calculus in the 12th. Precalculus is Related posts: How Much Does an Online Math Tutor Cost? Across the world, math is the key to understanding many... Best Online Calculus Math Tutor: How to Choose Calculus and math require tremendous background information, practice, and good... Online Algebra Math Tutor Many private and public high schools and colleges require students... Reviewing Six Online Math Tutoring Services - What’s the Best? Math is an exciting field of study that can lead...
Surrey Mathematics Research Blog The blog on research in mathematics at the University of Surrey
- Stanislaw Biber speaks at the XIX Conference of the Polish Magnesium Societyby Tom Bridges on October 20, 2025 at 3:56 pm
The XIX Conference of the Julian Aleksandrowicz Polish Magnesium Society was held 16-18 October at Potocki Palace (near Poznań, Poland). The theme was “Magnesium and other bioelements in medical, chemical, and natural sciences“. Stanislaw Biber was an invited speaker, and his talk “Dynamics of elements in soil — a mathematical approach at capturing the changes
- Quantum Sciences Research Retreat organised by Andrea Rocco and Alessandro Torrielliby Tom Bridges on October 10, 2025 at 2:56 pm
On Wednesday 8th October a one-day meeting on Quantum Sciences Research was hosted by the School of Mathematics and Physics and organised by Andrea Rocco and Alessandro Torrielli. The idea was to get members of the Quantum Sciences Group to speak about their latest research, and to invite others in the School whose research contains
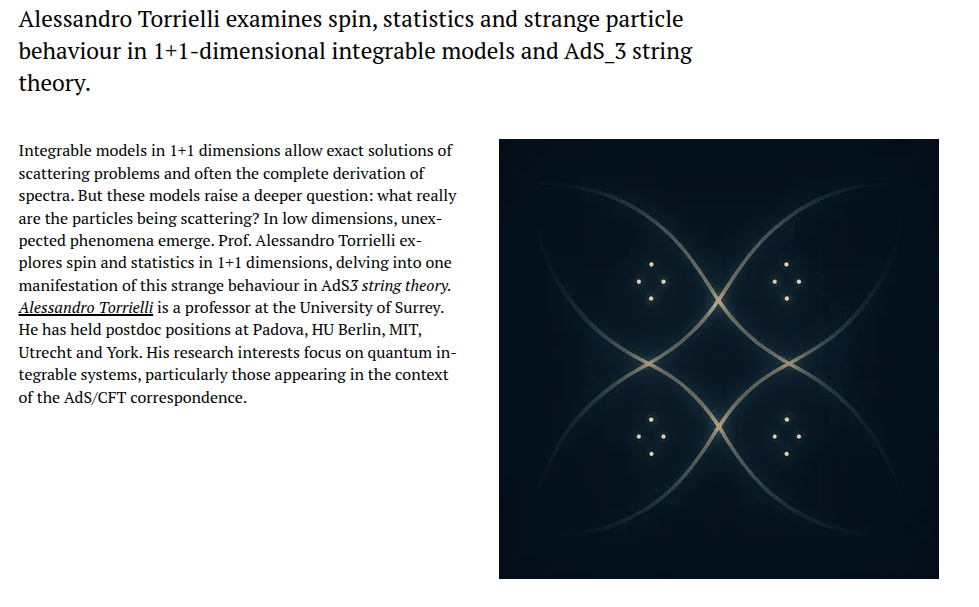
- Alessandro Torrielli gives a talk at the London Institute for Mathematical Sciencesby Tom Bridges on October 6, 2025 at 11:44 am
Alessandro Torrielli was in London on Friday 4th October to give a seminar at the London Institute for Mathematical Sciences. LIMS is hosted by the Royal Institution (link here). Alessandro gave a talk on “Integrable statistics” (link to event page here). A wine-reception was held in the Royal Institute afterwards, in a room with a
- Werner Bauer attends and gives a talk at the Firedrake 2025 workshop at Leedsby Tom Bridges on September 30, 2025 at 5:18 pm
Werner Bauer visited the School of Mathematics at the University of Leeds from 15-17 September for the 2025 version of the Firedrake workshop (link to workshop page). It is the tenth Firedrake user and developer workshop. Werner attended and gave a talk in Session 12 on “Fully implicit timestepping methods for the rotating shallow water
- Workshop on the interface between Chemistry and Mathematics organised by Cesare Tronciby Tom Bridges on September 30, 2025 at 10:11 am
On Thursday 2nd October, there was a one-day “Workshop on Mathematics and Chemistry“, held in the School of Mathematics and Physics, organised by Cesare Tronci. Funding has been provided by a grant from the Leverhulme Trust. The aim was to bring together different expertise in physics, applied mathematics, and computational/theoretical chemistry in an attempt to
Mathematics – Wolfram Blog News, Views and Insights from Wolfram
- Learning Decision Process Theory with a Wolfram Language Toolkitby Gerald H. Thomas on August 22, 2025 at 7:30 pm
In our daily lives, individuals, corporations and societies are constantly involved in making decisions. We hope to make optimal choices, especially when faced with recurrent decision processes. Thus we care about why and how our decision processes change over time. As a practicing engineer and an instructor in higher education, it is my opinion that
- What’s Up with Daylight Saving Time? A Brief History and Analysis with Wolfram Languageby Nick Lariviere on March 5, 2025 at 5:12 pm
In the next few days, most people in the United States, Canada, Cuba, Haiti and some parts of Mexico will be transitioning from “standard” (or winter) time to “daylight” (or summer) time. This semiannual tradition has been the source of desynchronized alarm clocks, missed appointments and headaches for parents trying to get kids to bed at the right time since 1908, but why exactly do we fiddle with the clocks two times a year?
- A Whole New Ball Game: Game Theory in Wolfram Language 14.2by Marc Vicuna on February 25, 2025 at 6:17 pm
Do you want to make optimal decisions against competition? Do you want to analyze competitive contexts and predict outcomes of competitive events? Do you need to elaborate strategies and plans against adversity and test the effectiveness of those strategies? Or are you simply an undergraduate student struggling to cope with a required course on game
- Master the Basics of Laplace Transforms in Just 15 Lessons with Wolfram Languageby Juan Ortiz on February 5, 2025 at 8:30 pm
The Laplace transform provides effective and easy means for solving many problems that arise in the fields of science and engineering. It is one of the main tools available for solving differential equations. For most of us, the first time we see it is in an introductory differential equations course. Wolfram Language provides an ideal
- Learn Complex Analysis Today with Wolfram Languageby Marco Saragnese on October 15, 2024 at 3:44 pm
Complex analysis is a versatile tool that is used extensively in science, engineering and other fields. It is also a beautiful topic in and of itself. Hence, a course in complex analysis is a standard part of the curriculum for physics and engineering students and a stepping stone for more advanced topics in mathematics. Wolfram
- Hypergeometric Functions: From Euler to Appell and Beyondby Tigran Ishkhanyan on January 25, 2024 at 5:35 pm
Hypergeometric series appeared in the mid-seventeenth century; since then, they have played an important role in the development of mathematical and physical theories. Most of the elementary and special functions are members of the large hypergeometric class. Hypergeometric functions have been a part of Wolfram Language since Version 1.0. The following plot shows the implementation
- Get Down to Business with Finite Mathematics in Wolfram Languageby John McNally on December 22, 2023 at 3:41 pm
“There is every reason to expect that the various social sciences will serve as incentives for the development of great new branches of mathematics and that some day the theoretical social scientist will have to know more mathematics than the physicist needs to know today.” —John G. Kemeny, first author of the original textbook on
- Don’t Be Discreet and Learn Discrete Mathematics with Wolfram Languageby Marc Vicuna on November 29, 2023 at 6:00 pm
“The spread of computers and the internet will put jobs in two categories. People who tell computers what to do, and people who are told by computers what to do.” — Marc Andreessen, inventor of the Netscape browser How is data organized in databases? Why are some computer programs faster than others? How can algorithms
- Learn Multivariable Calculus through Incredible Visualizations with Wolfram Languageby Tim McDevitt on November 6, 2023 at 3:57 pm
Multivariable calculus extends calculus concepts to functions of several variables and is an essential tool for modeling and regression analysis in economics, engineering, data science and other fields. Learning multivariable calculus is also the first step toward advanced calculus and follows single-variable calculus courses. Wolfram Language provides world-class functionality for the computation and visualization of